AP Physics 2 – Circuits and Capacitors Review Problems
1. A particular cylindrical resistor is intended to have a resistance of 1.5 kΩ and has length 1.0 cm and diameter 2.0 mm. (a) Determine the required resistivity of the material used to form the cylinder. (b) Given the resistivity of gold is 2.44 × 10–8 Ωm, how long a cylinder of the same diameter or what diameter the same length would be needed to create the same resistance?
2. The resistivity of the metal in a soup can is 1.0 × 10–7 Ωm. Suppose the top and bottom are cut out of a can with length 11 cm, diameter 7.5 cm, and thickness 0.20 mm (an empty cylinder, no ends). (a) What current passing from top to bottom would produce a measureable voltage of 1.0 mV? (b) Repeat for a current passing from one side of the can to the opposite side (across a diameter).
3. A steel cable of length 20.0 m and radius 1.2 cm is hit by lightning and carries a current of 5.0 kA. Given the resistivity of steel, 1.4 × 10–7 Ωm, determine the power generated in the cable – i.e. the rate at which it dissipates heat.
4. A certain pencil of length L has lead of radius r and resistivity ρ1 embedded in a wooden cylinder with inner radius r and outer radius 2r and resistivity ρ2. (a) Derive an expression for the overall resistance of the pencil if the ends are connected to a voltage source. (b) What ratio of resistivity would be needed so that the wood and the lead carry equal amounts of current?
5.
An experiment is done measuring
current through and potential difference across a Light-Emitting Diode (LED)
resulting in the following data showing five trials.
Current (μA) Voltage
(V)
9.4 1.676
13.6 1.689
1646 1.853
3150 1.892
4840 1.926
(a) Determine the apparent resistance of the LED for the first and last
trials. (b) Based on the data is it ohmic or nonohmic? Explain. (c) Assuming
it could be treated as ohmic for voltages below 1.6 V what would be the current
if attached to a 1.50 V cell? (d) Once beyond a certain voltage the LED
“lights up” and its behavior is somewhat ohmic in the sense it can be reasonably
modeled by an equation V = V0 + Ir, where V0
is an “activation voltage” and r is resistance. Determine values for V0
and r using the last two trials. (e) Based on this model what is the current
and apparent efficiency of the LED when operating on 2.00 V?
6. A battery labeled 9.0 volts is connected to a 33 Ω resistor and a current of 260 mA results. (a) What is the terminal voltage in this situation? (b) Internal resistance of what amount would explain the results?
7. Two resistors of resistance R and 2R are connected to an ideal battery. If connected in parallel both resistors have more power than if connected in series. Compare parallel to series: (a) By what factor does the power of resistor R increase? (b) By what factor does the power of resistor 2R increase? (c) By what factor does the overall power increase?
8. The illuminated incandescent bulb in a flashlight emits energy (heat and light) at a rate of 0.75 W. The battery consists of two cells, each with emf 1.50 V and internal resistance 0.800 Ω, stacked in series. (a) Given this information it is possible to determine the resistance of the bulb, but there are two valid solutions mathematically – find both. (b) Assuming the flashlight is designed to operate efficiently and provide light for as long as possible, which solution seems most likely? Explain.
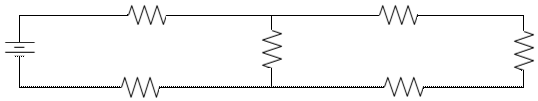
9.
Consider the circuit shown below
in which all of the resistors have equal resistance and are powered by an ideal
battery with voltage V. (a) Determine the voltage across each resistor
in terms of V. (b) There are three different currents in the circuit –
determine the ratio of these currents. (c) If one of the resistors is replaced
by a capacitor it could be charged to one of three different voltages –
determine these voltages in terms of V. (d) Determine the ratio of the
initial currents to the capacitor in these three different scenarios.
10. A capacitor of capacitance 0.60 F that has been previously charged to a voltage of 6.00 V is then used to partially recharge a battery with emf 3.70 V and internal resistance 1.10 Ω. (a) Determine the initial current into the rechargeable battery. (b) Explain why this current immediately decreases and over time approaches zero. (c) How much charge is ultimately “extracted” from the capacitor? (d) Determine the amounts of energy: lost by the capacitor, gained by the rechargeable battery, and wasted as heat.
11. Two capacitors of capacitance 220 μF and 440 μF are each charged up to a voltage of 12 V. The capacitors are then put into a different circuit with a 50.0 Ω resistor (and without a battery) in the manner that will produce the maximum amount of current. (a) Determine the maximum current through the resistor – how should the capacitors be connected to achieve this? (b) The lesser of the two capacitors reaches a state of zero charge when the current is what value? (c) Determine the final voltage of each capacitor and the amount of heat dissipated by the resistor given enough passage of time. (d) How can the two capacitors and one resistor be rewired at this point in order to completely release all of the stored energy? Explain.
12. An experiment is done creating a capacitor with two aluminum pizza pans of radius 23 cm separated by an air gap of 1.00 mm. The improvised capacitor is connected to a 6.0 V battery. (a) Determine the capacitance, charge on each pan, potential energy and electric field strength between the pans. (b) Repeat for a case where the space between the pans is filled with paper of dielectric constant 3.5. (c) Now suppose the battery is disconnected and then the paper is removed without disturbing or discharging the pans – determine the voltage, potential energy, and electric field strength.
Answers
1.
a. 0.47 Ωm
b. 190 km long (about 100 miles) or diameter 0.45 μm (about like a spider web)
2.
a. 4.3 A
b. 3.7 A
3. 150 kW
4.
a. ![]()
b. ρ2 = 3ρ1
5.
a. 180 kΩ, 398 Ω
b. The ratio of voltage to current decreases drastically and therefore it is
nonohmic. an ohmic device has a constant ratio of voltage to current.
c. 8.4 μA
d. 1.83 V, 20.1 Ω
e. 8.52 mA, 91.4%
6.
a. 8.6 V
b. 1.6 Ω
7.
a. 9
b. 9/4
c. 9/2
8.
a. 0.30 Ω or 8.5 Ω
b. The greater resistance 8.5 Ω makes more sense because less energy is
being wasted by the internal resistance of the cells and it will be more
efficient use of the energy stored, therefore lasting longer. (Strangely
either solution is possible to provide the same amount of light ouput!)
9.
a. two resistors nearest the
battery have voltage 4V/11, three resistors farthest from the battery
have voltage V/11, and the middle resistor has voltage 3V/11
b. currents in the same order have the ratio 4 : 1 : 3
c. V, V/3, 3V/5 (replacing a resistor in the same order)
d. currents in same order have ratio 4/7 : 1/8 : 1/2
10. a. 2.1 A
b. The current causes the amount of charge in the capacitor to decrease, which
also causes its voltage to decrease. With less capacitor voltage there is less
potential difference driving the current.
c. 1.4 C
d. 6.7 J, 5.1 J, 1.6 J
11. a. 0.48 A – connect the capacitors in series, positive
plate of one to negative plate of the other
b. 0.12 A
c. 4.0 V; 42 mJ
d. If the two capacitors are wired in parallel, positive plate to positive
plate, the current through the resistor will discharge both capacitors. The
voltage of each will drop from 4 V to 0 V in synchronization with the greater
capacitor providing 2/3 of the current and the lesser providing 1/3 of the
current.
12. a. 1.5 nF, 8.8 nC, 26 nJ, 6.0 kN/C
b. 5.1 nF, 31 nC, 93 nJ, 6.0 kN/C
c. 21 V, 320 nJ, 21 kN/C