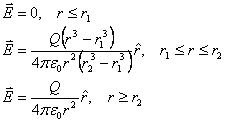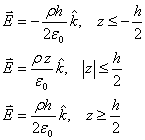AP Physics Assignment - Electric Flux and Potential

AP Physics Assignment – Electric Flux and Potential

Reading Chapters 24 and 25

Objectives/HW

	The student will be able to:	HW:
1	Define and apply the concept of electric flux and solve related problems.	1 – 5
2	State and apply Gauss’s Law and solve related problems using Gaussian surfaces.	6 – 17
3	Calculate work and potential energy for discrete charges and solve related problems including work to assemble or disassemble.	18 – 25
4	Define and apply the concept of electric potential and solve related problems for a discrete set of point charges and/or a continuous charge distribution.	26 – 32
5	Use the electric field to determine potential or potential difference and solve related problems.	33 – 36
6	Use potential to determine electric field and solve related problems.	37 – 39
7	State the properties of conductors in electrostatic equilibrium and solve related problems.	40 – 46

Homework Problems

1. A uniform electric field of 25 kN/C is directed parallel to the z-axis. A square of sides 5.0 cm is submersed in this field and initially located in the xy-plane. (a) Determine the electric flux for the square. (b) Repeat if the square is rotated 40.0° about the x-axis. (c) Repeat if the square is moved again and this time its normal is parallel to the x-axis.

2. Two otherwise identical adjacent houses have different roofs. The roof of House #1 is steeper (greater slope) than the roof of House #2. (a) Compare the total amount of rainwater that is collected by the gutters of each house during a storm – which is greater or is it equal? Explain. (b) Suppose the rain falls at an angle due to wind. Would this affect the result? Explain.
Hint: This is a flux problem – the rain is like the electric field and the roof is the area. The total water collected is proportional to the flux.

3. Two circular parallel metal plates are oppositely charged with q = ±1.0 nC. The two plates each have a radius of 5.00 cm and are separated by 1.00 mm. (a) Estimate the electric flux for a circular area of radius 5.00 cm sandwiched between the two plates. (b) Repeat for a circular area of radius 5.00 meters – the result would be essentially the same. Explain why this would be the case.

4. Both charges of an electric dipole are located on the positive z-axis. The two charges are just “above” the xy-plane. The electric flux for the entire xy-plane would be zero even though the flux for certain regions of the xy-plane would not be zero. (a) Explain how this is possible. (b) Explain why the net flux for the entire plane would be precisely zero.

5. A certain electric field has a uniform direction in the positive z-direction but a magnitude that varies according to E = 20.0 x², where E is in N/C and x is in meters. (The field does not vary in the y-direction.) Determine the electric flux for a rectangle in the xy-plane with one corner on the origin and the opposite corner at coordinates (2.0 m, 3.0 m).

6. A point charge of +8.0 nC is located at the center of a sphere of radius 10.0 cm. (a) Find the electric flux for the sphere. (b) Suppose a second charge of −8.0 nC is then brought to a location 20.0 cm away from the first – what is the electric flux for the sphere? (c) With the second charge in place, compare the flux for two halves of the sphere – the hemisphere nearest the negative charge and the hemisphere opposite that. Which hemisphere would have the greatest flux and why? Hint: sketch the sphere and the field of the dipole.

7. A point charge of −3.0 nC is located at the center of a cubical box of sides 20.0 cm. (a) Find the electric flux for the entire box. (b) Find the electric flux for one side of the box.

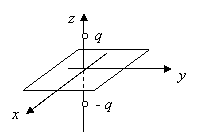
8. Two point charges, q and −q, are located on the z-axis at z = a and z = −a as shown in the diagram below. Find the net flux due to these charges through a square surface of side 2a, located in the xy-plane and centered on the origin. Hint: consider the previous problem and the superposition principle.

9. A certain charge distribution is located in a rectangular box (like a shoe box) standing on end. Suppose you (magically) determine the electric flux for each surface of the box: the square top = 115 N m²/C, each rectangular side = 95 N m²/C, the square bottom = −15 N m²/C. (a) What must be the total amount of charge inside the box? (b) Is all of the charge in the box of the same type (positive or negative)? If not, of which is there more and how much more? (c) Make a sketch of the box, showing roughly the electric field at the surfaces. (d) Based on your sketch (and your imagination) make an intelligent guess as to what type of charge distribution is inside the box.

10. A hollow metallic sphere of radius 10.0 cm is given a charge of 2.0 μC. At the center of the sphere is a point charge of −1.5 μC. (a) Find the electric field 5.00 cm from center. (b) Find the electric field 20.0 cm from center. (c) Sketch a graph of electric field vs. distance from center.

11. A total charge Q is placed on a hollow spherical shell. The charge is distributed uniformly throughout the volume of the shell from inner radius r₁ to outer radius r₂ (there is no charge in the hollow part of the shell). (a) Find the electric field as a function of r from the center. (b) Sketch a graph of the function – are there any discontinuities?

12. A photocopying machine employs a charged cylindrical “drum” of length 40.0 cm and radius 5.00 cm. The field just above the surface of the drum is 225 kN/C, radially outward. (a) Estimate the surface charge density for the drum. (b) Estimate the total charge on the surface of the drum. (c) If a different model photocopier uses a drum of length 30.0 cm and radius 3.5 cm, what must be the total charge on its surface to produce the same electric field near the surface?

13. In a certain factory operation powder is sent through a long pipe of radius 4.00 cm. The particles in the powder typically become charged in the factory processes. (a) If the charge density of the powder is a uniform 1.0 mC/m³, what is the maximum electric field that occurs inside the pipe? (b) A spark may occur if the field reaches 3.0 MN/C – at what charge density would this occur?

14. Charge is distributed nonuniformly throughout a long cylindrical volume of radius R. The charge is denser the closer to the edge of the cylinder such that the charge density is given by ρ = br, where b is a constant. Determine the electric field within and without the cylinder.

15. A planar “slab” of charge parallels the xy-plane. The thickness of the slab is h; it extends h/2 above and below the xy-plane. The charge has a uniform density per volume ρ. Assuming the slab “stretches to infinity” find the electric field within and without the slab as a function of z.

16. A charged wire with charge per length λ = −2.2 × 10⁻⁸ C/m extends parallel to a charged planar surface with charge density σ = −1.5 × 10⁻⁷ C/m². The wire is 6.0 cm from the surface. (a) Make a sketch of the electric field seen in cross section (a plane perpendicular to the wire). (b) Determine the electric field at a point halfway between the wire and the surface. (c) Solve for the location(s) where the electric field would be zero.

17. Suppose it is desired to use Gauss’s Law to solve for the electric field surrounding two equal point charges separated by a relatively small distance. (a) Sketch the field and attempt to draw an appropriate Gaussian surface. (b) What must be true of the surface in order for it to be suitable for finding the field? (c) Do you think such a surface exists? Explain.

18. Two pith balls are separated by 10.0 cm. The pith balls have charge: q₁ = 3.0 nC, q₂ = 2.0 nC. (a) Find the potential energy of this arrangement. (b) In order to increase the potential energy of the same two pith balls by 0.30 μJ what must be done to the arrangement? (c) Repeat (a) and (b) with : q₁ = 3.0 nC, q₂ = −2.0 nC.

19. Two opposite point charges, ±5.0 nC are separated by 2.0 cm. (a) Find the potential energy of this arrangement. (b) Find the net work that must be done by external force in order to increase the separation of the charges to 10.0 cm. (b) What additional work would be necessary to separate the two charges to a great distance?

20. Consider the potential energy of two point charges separated by a certain distance. (a) If the two charges have the same sign, what action will increase the potential energy – increasing the separation or decreasing the separation? Explain. (b) If the two charges have opposite signs, which action will increase the potential energy? Explain.

21. (a) Determine the amount of work necessary to assemble a set of point charges, 2q, 3q, and −q into an equilateral triangle of side s. (b) Repeat, but this time suppose the three charges are assembled into a line with the negative in the center and the two positives a distance s on either side. (c) Considering the two arrangements, how much energy could be “released” by rearranging from one to the other? Which arrangement should be the “starting point” if energy is to be released?

22. Four point charges, each equal to 75 nC, are placed at the corners of a square with sides 15.0 cm long. (a) Find the potential energy associated with one of these charges. (b) Find the potential energy of the entire system.

23. A Van de Graaff generator can be used as a particle accelerator. Suppose the Van de Graaff sphere has charge −2.0 μC and radius 0.20 m. An electron starts near the surface and accelerates away from the sphere. (a) Find the kinetic energy and speed of the electron when it is 0.30 m away from the center of the sphere. (b) What is the maximum speed to which the electron could be accelerated by the sphere?

24. In the classical model of the atom the electron is thought to orbit the nucleus like a planet orbiting the sun. Apply this model to a monatomic hydrogen atom, in which a proton is orbited by an electron at a certain radius depending upon its energy level. (a) Derive an expression for the total energy of the orbiting electron. (b) If an electron drops from level n = 2 to level n = 1, the atom emits a photon with a certain amount of energy. Determine this energy given the radii of the two orbits: r₁ = 5.29 × 10⁻¹¹ m, r₂ = 2.12 × 10⁻¹⁰ m.

25. Two charged pith balls are connected by a silk thread that is 90.0 cm long. The masses and charges of the pith balls are: m₁ = 0.10 g, m₂ = 0.15 g, q₁ = 2.0 nC, q₂ = 3.5 nC. The pith balls and thread are floating in outer space, initially at rest (for some strange reason). (a) The thread suddenly breaks – what is the initial acceleration of each ball? (b) What will be the eventual speed of each ball as it flies off in space?

26. Calculate the electric potential at distances of 5.0 cm and 10.0 cm away from a pith ball with charge −3.0 nC.

27. Calculate the electric potential at distances of 15.0 cm and 30.0 cm away from a pith ball with charge +3.0 nC.

28. A point charge q₁ = 10.0 nC is located at the origin and another point charge q₂ = −5.0 nC is located at position r = 6.0 i cm, relative to the origin. (a) Find the potential at r = 3.0 i cm. (b) Find the potential at r = 3.0 j cm. (c) Find the potential at r = (6.0 i + 3.0 j) cm. (d) At what position(s) along the x-axis would the potential equal zero?

29. Sketch the electric field and equipotential lines surrounding the charges in the previous problem and note the approximate location(s) where V = 0 and where E = 0. Using different colors indicate which regions are positive electric potential and which are negative.

30. A thin rod is given a charge Q distributed evenly along its length L. Find the electric potential along the axis of the rod as a function of x, the distance from one end of the rod.

31. Suppose the rod from the previous problem has properties: L = 30.0 cm, and Q = −90.0 nC. (a) Determine the electric potential at x = 5.0 cm. (b) At what position along the axis would the potential be half its value at x = 5.0 cm? (c) Calculate the potential at x = 20.0 m and the potential 20.0 m away from a point charge of the same amount. Compare – should these values be about the same?

32. Suppose a proton is released from rest at x = 20.0 m from the rod in the previous problem. What would be its speed upon arriving at x = 5.0 cm? Assume it travels through a vacuum.

33. In a classroom experiment a pith ball of mass 0.12 g is held at a position r_A = 0.50 m above the center of a Van de Graaff generator. The pith ball is then released and falls to a position of r_B = 0.30 m before “rebounding” due to repulsion from the generator. The field of the generator in this region is given by E = 95/r^1.5 downward, where E is in kN/C and r is in m. (a) Determine the potential difference, V_B – V_A, between the two positions. (b) What must be the charge on the pith ball to account for its behavior?

34. Two parallel metal plates, each with an area of 50.0 cm², are separated by 1.00 mm. Suppose the plates are given opposite charges of ± 5.0 nC. (a) What is the potential difference between the two plates? (b) If the separation of the plates is increased to 3.00 mm without changing the amount of charge, what becomes of the potential difference?

35. A total charge of Q is distributed uniformly throughout a sphere of radius R. Determine the potential at the center of the sphere.

36. A point charge q = +7.0 nC is located on the z-axis at z = 3.0 cm. The xy-plane has a uniform surface charge σ = −5.5 μC/m². Determine the potential difference, V_B – V_A, between positions: r_A = 6.0 k cm and r_B = (1.0 i + 3.0 k) cm.

37. A certain electron gun in a picture tube consists of oppositely charged parallel plates with a potential difference of 22 kV. (a) With what speed can this gun “fire” electrons? (b) If the two plates are 0.90 cm apart, what is the electric field in the space between? (c) Repeat both questions if the plates are only 0.45 cm apart but the potential difference is the same.

38. Use the potential for the thin rod along the axis (the function found in problem #30) to solve for the electric field as a function of x, the distance from one end of the rod. Compare to the same function found previously by Coulomb’s Law and integration.

39. In a particular region of space the electric potential can be modeled by V = y²/4, where y is in meters and V is in volts. (a) Make a sketch of the xy-plane from y = -5 m to y = +5 m and show nine equipotential lines where V = 0, 1, 2, 3, and 4 volts. (b) Determine the electric field in the region as a function of y (include direction). (c) Add electric field lines to the sketch of equipotentials. (d) What distribution of charges might create such a region? Add to your sketch.

40. A metal box is given a charge of -5.0 μC. The cubic box encloses a certain volume of space. (a) Where does the negative charge reside – on the inside surface, the outside surface, or throughout the metallic volume? (b) Is the charge spread uniformly? If not, where is it concentrated? Explain. (c) The box is temporarily opened and a point charge of +1.5 μC is placed inside and the box is closed again – without changing the net charge on the box. How does this affect the distribution of charge in the metal of the box? Explain. (d) Would the field outside the box depend upon where the point charge is located inside the box? Explain.

41. In the operation of a Van de Graaff generator charge is collected by a metallic “brush” near the center of hollow metal “globe”. The charge enters the globe through a small hole in one side, carried by a rubber belt. (You can find a diagram in your book.) (a) Explain why it is necessary to have the collection point on the inside of the globe in order to build up a substantial charge upon it. (Why wouldn’t it work as well to add charge to the side of the globe?) (b) Describe the journey of a single electron beginning with a position on the belt outside the globe and ending with a position of electrostatic equilibrium in the metal of the globe. What path does it follow? What forces act upon it?

42. (a) Determine an expression for the amount of potential energy associated with charging a metallic sphere of radius R to a final charge amount Q. Hint: this is basically the work to assemble the charge – as the total amount of charge on the sphere increases it becomes “harder” to add charge to its potential increases . (b) Does it matter whether or not the sphere is hollow? Does it matter to which part of the sphere the charge is conducted? Explain.

43. A long, straight metallic pipe of radius R has a uniform charge per area σ. Determine the electric field and electric potential for regions inside and outside the pipe. Hint: taking the pipe to be infinitely long, the potential of the pipe itself must be what, considering our usual reference?

44. A copper sphere of radius 8.00 cm is initially charged to +75.0 nC. The sphere is then connected by a long thin wire with another copper sphere of radius 16.0 cm located on the other side of a large room. (a) Assuming that the spheres are far enough apart to be considered isolated (and that very little charge remains on the wire), what will be the amount of charge on each sphere when electrostatic equilibrium is attained? (b) What will be the surface charge density on each sphere? Hint: how should the potential at the surface of each sphere compare?

45. Two parallel metal plates are connected by wires to a battery, as shown in cross section below. The potential difference of the battery’s terminals is: V_B – V_A = +9.00 V. The plates each have area 0.0400 m² and are separated by 1.50 mm. (a) What is the potential difference between points A and C? between points B and D? How do you know? (b) What is the potential difference : V_C – V_D = ? (c) Sketch the two plates and show electric field lines and equipotential lines in the region between. (d) What is the magnitude of the electric field between the plates? (e) Determine the net amount of charge on each plate.

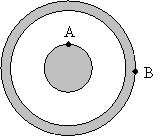
46. A solid metal sphere of radius 5.00 cm is located at the center of a hollow metal sphere with inner and outer radii 12.0 cm and 14.0 cm. Each sphere has a net charge; the net charge of the outer sphere is -70.0 nC. Between points A and B shown in the diagram below, the potential difference is: V_B – V_A = -8.50kV. (a) Determine the net charge on the inner sphere. (b) Determine the potential at the center of the inner sphere, relative to infinity. (c) What is the surface charge density on the outside surface of the outer sphere?

Selected Answers