AP Physics Resistance and Resistivity Lab
Purpose
The goal of this lab exercise is to measure electric current through wire and determine the relations between voltage, current, resistance, and the physical properties of the wire.
Overview
The basic procedure of this experiment is to send a known current through a wire and measure the electric potential difference (or voltage) across a certain length. This entails connecting a wire to a power source. This is an “unusual” thing to do! Because a wire is a conductor, large current can and will occur, depending on the voltage of the power source. For example, if you connect a wire to an ordinary battery a large current ensues, and the wire will rapidly heat up and quickly discharge the energy stored the battery – ruining it in the process. It is important to use caution with the adjustable power supply used in this experiment. Always start with the controls turned all the way down to zero and then slowly turn it up until a desired current is obtained. If this is not done damage may occur to the equipment!
Procedure
Start with the smallest diameter copper wire. Lay the wire out along a meter stick and place a cork pad under each end so that the wire is elevated slightly above the stick. Use clamps to attach the wire to the stick, sandwiching the cork between the wire and the stick. Use jumper cables to connect a multimeter, functioning as an ammeter – connect the positive terminal of the power supply to the terminal labeled 10A on the meter, connect the COM terminal of the meter to one end of the wire. Connect the other end of the wire to the negative terminal of the power supply. This forms a single continuous loop through which a certain current can flow. The current will be the same throughout the loop.
Now prepare another multimeter, functioning as a voltmeter. Plug in two alligator clip cables to the V terminal and the COM terminal. Use the alligator clips to connect to the wire at two points along the meter stick. The scale on the meter stick may be used to determine the length of wire being tested. Note that the presence of this voltmeter has a very negligible effect on the current flowing through the loop formed by the power supply, ammeter, and wire sample.

The same circuit shown schematically would appear as follows:
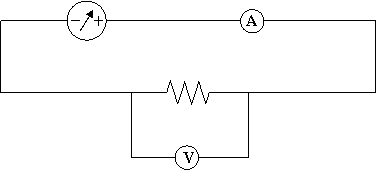
Using the ammeter:
Check the meter for proper connections to the 10A and COM terminals and then switch the selector to the 10A setting. In this condition, the meter measures the conventional positive current entering through the 10A terminal and exiting the COM terminal. The reading will be negative if the current is opposite. The maximum measurable current is 10 amperes.
Using the voltmeter:
Check the meter for proper connections to the V and COM terminals and then switch the selector to the V setting. In this condition, the meter measures the potential difference of the V terminal relative to the COM terminal. The reading will be negative if the potential is higher at the COM terminal than at the V terminal. In this experiment the values will typically be measured in millivolts, which shows as mV in the display.
Using the power supply:
Before turning it on, adjust both control knobs all the way to zero (fully counterclockwise). Turn the unit on and then turn the current knob up first and then the voltage knob. Do not exceed a current of 3.3 amperes! In order to get a specific current use the following procedure: adjust both knobs until there is greater current than needed and then back off on the current knob until you get the desired number of amperes. The current limited indicator will come on but this is normal and simply means that the current cannot exceed a certain value. Note: the voltage and current shown by the built-in meters of the power supply should be considered ONLY as an approximate reference – the values shown by the multimeters should be more accurate.
Part A – Voltage vs. Current
Use copper wire with diameters of 18 gauge and 16 gauge. Note: 16 gauge is a greater diameter than 18 gauge – greater gauge indicates thinner wire. The length represents the distance along the wire between the two connections of the voltmeter (not the total length of the wire). Measure the resulting voltage for currents of approximately 0.50 A through 3.00 A, steps of 0.50 A. It may be difficult or nearly impossible to set the current to an exact value – if you cannot get it exactly where you want it just get it close and record whatever the meter is reading.
Part B – Resistance vs. Length
Use the wire of unknown metal. For this part of the lab a different procedure is used. Set the current as close as possible to 1.000 A. Then adjust the measured length from 80.0 cm to 20.0 cm in steps of 10.0 cm. For each length note the voltage and make sure that the current is still 1.000 A. Because the number of ohms resistance is equivalent to the number of volts per 1 ampere, the reading of the voltmeter may be interpreted as equivalent to the resistance measured in ohms. This is only true if the current is precisely 1.000 ampere!
Analyses
1. Create appropriate, well-labeled, high quality graphs based on the data tables. For the copper wire data it is appropriate to place multiple data sets on one graph – for example, all three sets of data for 18 gauge could be placed on one graph and then all three sets of data for 16 gauge could be placed on another. Or if you prefer you could put all six sets on one “monster” graph. However it is done, you need to use different colors and/or symbols and include a key or legend. The data for the unknown metal must occupy a separate graph. Include an appropriate curve-fit and corresponding equation for each set of data (seven curve fits).
2. Resistance is defined as the voltage across a wire (or other material) divided by the current through it. Based on this definition, the resistance is equivalent to one of the coefficients in the curve-fit equation for each particular length and diameter of wire. Use this fact to complete the table showing the resistance of each length and diameter of copper wire (this should be six values).
3. Determine the cross-sectional area (the area of a circle in m2) for each wire sample. Then divide the length (in m) by the cross-sectional area. This ratio, length per area, is a large number (measured in m-1). Complete the table showing resistance versus length per area for all six sets of copper wire data. Make a graph of these six values and find the line of best fit and corresponding equation.
Questions
1. What type of relation exists between voltage and current? How does your data support this? Be specific and refer to the graphs.
2. Draw conclusions about resistance based on your data, tables, and graphs: (a) How does resistance appear to depend on length? (b) How does resistance depend on diameter? Be specific and support your answers with your results
3. The slope of resistance versus length per area represents an inherent property of copper. What is it called? Consult a physics text and find the accepted value and determine the percent error.
4. Consider the graph for the unknown metal. Use the result to determine the resistivity of this sample. Show all work. What is the most likely element from which this wire is made?. Consult a physics book and a table of such values to find out.
5. Discuss error.
Data
Part A – Voltage vs. Current for Copper Wires
|
|
18 Gauge Copper Wire |
16 Gauge Copper Wire |
||
|
|
Diameter = |
Diameter = |
||
|
Length (cm) |
Current (A) |
Voltage (mV) |
Current (A) |
Voltage (mV) |
|
80.0 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
60.0 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
40.0 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Part B – Resistance vs. Length for Wire of Unknown Metal
|
Diameter = |
|
|
Length (cm) |
Resistance (mΩ) |
|
80.0 |
|
|
70.0 |
|
|
60.0 |
|
|
50.0 |
|
|
40.0 |
|
|
30.0 |
|
|
20.0 |
|
Analyses
Resistance Determined from Curve-Fit Equations:
|
|
18 Gauge Copper Wire |
16 Gauge Copper Wire |
|
|
Diameter = |
Diameter = |
|
|
Area = |
Area = |
|
Length (cm) |
Resistance (mΩ) |
Resistance (mΩ) |
|
80.0 |
|
|
|
60.0 |
|
|
|
40.0 |
|
|
Resistance of Copper Wire as a Function of Combined Geometric Properties:
|
Length per Area (m-1) |
Resistance (Ω) |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|