AP Physics Assignment - Kinematics
Reading Physics for Scientists and Engineers – Chapters 1 and 2
Objectives/HW
|
|
The student will be able to: |
HW: |
|
1 |
Define, distinguish, and apply the concepts: distance, displacement, position. |
1, 2 |
|
2 |
Define, distinguish, and apply the concepts: average speed, instantaneous speed, constant speed, average velocity, instantaneous velocity, constant velocity. |
3 – 9 |
|
3 |
Define, distinguish, and apply the concepts: average acceleration and instantaneous acceleration, and constant acceleration. |
10, 11 |
|
4 |
Analyze a graph of distance, position, or displacement as a function of time in order to determine speed and/or velocity. |
12 – 14 |
|
5 |
Analyze a graph of speed or velocity as a function of time in order to determine distance, position, displacement, and/or acceleration. |
15 – 19 |
|
6 |
State the displacement and velocity relations for cases of constant acceleration and use these to solve problems given appropriate initial conditions and values. |
20 – 33 |
|
7 |
State and use the conditions of freefall, including the value of g, to solve associated problems. |
34 – 41 |
|
8 |
Measure and analyze data for a moving object and produce appropriate graphs including line or curve of best fit. |
lab |
|
9 |
Evaluate error, deviation, accuracy, and precision in experimental results. |
lab |
Homework Problems
1. An airplane takes off 550 miles north of K-town and undergoes a displacement of 225 miles north to its first stop. It then undergoes another displacement of 880 miles south to its final destination. (a) Determine the position of the first stop relative to K-town. (b) Find the net displacement of the airplane from its initial position to its final destination. (c) Find the position of the final destination relative to K-town. (d) Find the total distance traveled by the airplane.
2. Explain how it would be possible for a car to travel a distance of thousands of miles and yet have a displacement of zero.
3.
At
precisely 1:00 pm a GPS system in a car indicates that it is 350 km east
of
4. Let’s compare some speeds: car on interstate = 130 km/h (80 mph), sound = 343 m/s, light and radio waves = 299792458 m/s. (a) Determine the distance each travels in 1.28 s (which is the time for light to travel from Earth to the Moon). (b) Determine the amount of time for each to travel a distance of 78.4 Gm (the closest approach of Mars to the Earth).
5. Two cyclists have a race to the top of a hill and back – 550 m up and 550 m back down. Biker A has a speed of 8.0 m/s going up the hill and 16 m/s coming down. Biker B has a speed of 6.0 m/s going up and 18 m/s back down. (a) Which biker wins the race and by how many seconds? (Or is it a tie?) (b) What is the average speed of the winning biker? (c) What is the average velocity of the losing biker?
6. How fast is that car going that just passed you? Start counting just as the car passes, note where the car is on the road as you reach ten, keep counting until you reach the same place on the road. Divide the two numbers and multiply by your own speed. For example, suppose you are going 60 mph, a car passes you and you start counting. As you reach ten it goes under a bridge up ahead and as you reach fifteen you go under the same bridge. The car that passed you then has a speed given by: (15/10)×60 mph = 90 mph. (a) Explain specifically or show algebraically why this works. (b) Are there any circumstances under which this would not work? Explain.
7. A 10.0 m long truck traveling at 125 km/h passes a 3.00 m long car traveling at 115 km/h. How much time does it take for the truck to pass? (the time from when the front of the truck is even with the back of the car until the back of the truck is even with the front of the car)
8. Speed and velocity have similarities and differences. (a) Suppose an object’s average speed over a certain interval has the same magnitude as its average velocity over the same interval – what must be true of the object’s motion for this to happen? (b) Suppose average speed and average velocity have different magnitudes – how does this happen? (c) An object’s instantaneous speed always has the same magnitude as its instantaneous velocity – explain why this must be so in light of the mathematical limits that define these quantities.
9. When a jet passes by at a high altitude you see it before you hear it. This is because light and sound travel at different speeds while traveling the same distance. Similarly when an earthquake occurs waves of different speeds travel equal distance to a seismograph. Let v1 and v2 be the wave speeds (v1 > v2) and Δt be the difference in arrival time. (a) Derive an equation for finding the distance to the source of the waves in terms of these variables. (b) Calculate the distance to a lightning strike given: v1 = 3.00 × 108 m/s, v2 = 343 m/s, Δt = 5.0 s. (c) Calculate the distance to the epicenter of an earthquake given: v1 = 5300 m/s, v2 = 3200 m/s, Δt = 5.0 s.
10. At t = 0, a Tesla Model 3 accelerates from rest at maximum rate and reaches the following speeds: 26.8 m/s (60 mph) at t = 3.1 s, 44.7 m/s (100 mph) at t = 8.2 s, and 58.1 m/s (100 mph) at t = 16.2 s. Find the average acceleration rate for the following intervals: (a) from 0 to 60 mph, (b) from 60 to 100 mph, and (c) from 100 to 130 mph. (d) Describe how the acceleration of the car varies with respect to the speed of the car..
11. A car is traveling at constant speed with the cruise control set for 35.0 m/s. As the car encounters a hill the speed drops to 32.0 m/s before the cruise control “kicks in” to return it to the original speed. If the cruise control causes the car to accelerate 0.80 m/s2 how much time does it take to get back to 35.0 m/s?
12.
The
following graph shows the motion of a skydiver. (a) Find average velocity
during the 12 s shown on the graph. (b) Find the speed at t = 2 s.
(c) Find the maximum speed. (d) Does the skydiver attain a terminal
velocity? Explain. (e) Calculate the value of t and the
speed of the skydiver at the instant he hits the ground (zero elevation).
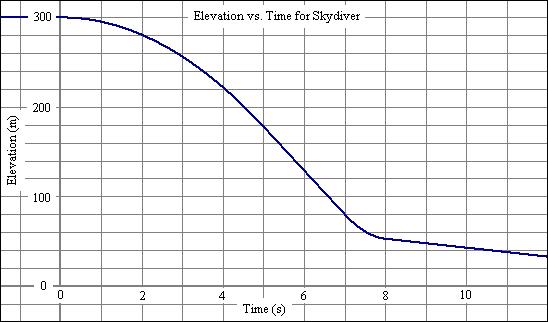
13. Make a careful sketch of the speed vs. time graph for the skydiver in the previous problem. At each inflection point label time and speed on the axes. Assume that whenever the speed is changing it does so at a constant rate.
14. Make a careful sketch of the acceleration vs. time graph for the skydiver – again label values at inflection points.
15.
The
following graph shows the motion of a 2007 Mini Cooper as it is tested for its
ability to accelerate and brake. The car was moving southward during the
test. (a) Determine its average acceleration from zero to 60 mph.
(b) Find the acceleration at t = 6.0 s. (c) Find the acceleration
at t = 20.0 s. (d) Find the acceleration at t = 40.0
s. (e) Find the acceleration at t = 54.0 s. (f) Determine
the maximum southward rate of acceleration.

16. Make a careful sketch of the acceleration vs. time graph for the car in the previous problem. At each inflection point label time and acceleration on the axes.
17. Based on its speed vs time graph, determine the distance traveled by the Mini Cooper during each of the following intervals: (a) from t = 0 to 30 s, (b) t = 30 to 50 s, (c) t = 50 to 60 s.
18. Make a careful sketch of the distance vs. time graph for the car in the previous problem. At each inflection point label time and distance on the axes.
19. Consider a person playing on a trampoline. Let t = 0 s as the person reaches the highest point of a particular jump from the trampoline. From this point on, the person simply stands straight without bending legs and falls onto the trampoline and rebounds into the air. The person falls from t = 0 to 0.50 s, contacts the trampoline from t = 0.50 s to 0.80 s, and rises from t = 0.80 to 1.20 s before falling again. (a) Make a graph of the person’s velocity vs. time from t = 0 to 1.60 s by assuming the acceleration is 10 m/s2 downward whenever the person is in the air. (b) Use the graph to determine the distance the person falls before touching the trampoline. (c) Use the graph to determine the amount that the trampoline is stretched. (d) Explain why it is not possible for the upward acceleration of the trampoline to be constant based on the results of this problem.
20. Starting from rest a cyclist coasts down a long hill with a uniform acceleration of 0.50 m/s2. At a particular point the cyclists notes his speed is 5.0 m/s. (a) How far had he rolled at that point? (b) How far does the he move in the next 10.0 s?
21. In 2001, Kenny Berstein’s dragster completed the Ľ-mile, a distance of 402.3 m, in 4.477 s. Assuming a constant acceleration determine: (a) final speed and (b) rate of acceleration.
22. The actual final speed of the dragster from the previous problem was 332.18 mph or 148.50 m/s. (a) Explain the discrepancy with the calculated speed. (b) Sketch a speed vs. time graph showing both the motion assumed in the previous problem and the motion as it most likely occurred in reality. (c) Repeat for distance vs. time. (d) Repeat for acceleration vs. time.
23. A bullet fired from a .357 magnum pistol has a speed of 410 m/s just as it leaves the barrel (this is called “muzzle velocity”). If the barrel is 11 cm long, find: (a) the rate of acceleration of the bullet, and (b) the time it takes to move through the barrel.
24. A typical car can decelerate at around 9.00 m/s2 when the braking is maximized. (a) Determine the stopping distance for a car initially traveling 30.0 m/s. (b) At what initial speed would the stopping distance be doubled from the previous result?
25. A Honda Fit can decelerate from 26.8 m/s (60 mph) to 0 in a space of 39.9 m and from 35.8 m/s (80 mph) to 0 in a space of 71.9 m. (a) Determine the rate of deceleration for each case. (b) Estimate the distance required to stop the car starting with a speed of 44.7 m/s (100 mph).
26. A certain car has acceleration 7.00 m/s2 when in first gear and 3.00 m/s2 when in second gear. Starting from rest the car travels 45.0 m in 4.00 s. Determine the speed at which the driver shifted gears (assuming this happens instantaneously).
27. While investigating an accident you measure skid marks that are 15 m long leading to the edge of a cliff. A typical car has a deceleration rate of 7.8 m/s2 while skidding. Based on where the car crashed below it is determined that it left the edge of the cliff at a speed of 20.0 m/s. Find the initial speed the car had at the beginning of the skid marks.
28. Consider a moving object with speed v that undergoes a constant acceleration of magnitude a that causes it to reverse direction. (a) Show algebraically that the distance traveled while slowing from v to 0 is equal to the distance traveled while going from 0 to v (in the opposite direction). (b) Sketch the object’s position vs. time and velocity vs. time graphs.
29. A car on an incline rolls backward as the driver takes his foot off the brake, hits the gas pedal and lets out the clutch. Suppose the car accelerates downhill at 2.00 m/s2 for 0.30 s and then upward at 3.00 m/s2 once the clutch engages. (a) Determine how far downhill the car rolls before moving forward. (b) Where is the car 1.0 s after it begins to move?
30. A simple model of sprinting is to assume a constant acceleration from rest followed by a constant speed. The world’s best sprinters can achieve a fairly uniform speed of 11.5 m/s as they complete the 100 meter dash in about 9.90 s, 0.15 s of which is typically reaction time to the start signal. (a) Determine the acceleration of a world class sprinter based on this info. (b) What percent of the race’s distance is spent accelerating? (c) What percent of the race’s time is spent accelerating?
31. How fast is the human
hand? Mr. M times his hand moving rapidly up and down. It takes
0.16 s for his hand to move 0.61 m from one extreme to the other. Assume
that acceleration and deceleration are both uniform and equal in
magnitude. (a) Find the maximum speed. (b) Find the acceleration
rate. (c) This acceleration must be exceeded in reality. Can the
same be said for the speed? Explain and discuss. Hint: a speed vs.
time graph is helpful.
(Try timing your own hand! – Move it up and down a known distance 10 times,
divide the total time by 20 to get the time for one “trip” through the
distance. Repeat the above calculations. Is your hand faster than
mine?)
32. Suppose a car is to travel a distance d between two stop signs, coming to a complete stop at each sign. (a) What is the smallest amount of time in which a car can do this in terms of its maximum acceleration rate, a1, and its maximum deceleration rate, a2? (b) Apply the result to the following values: d = 100.0 m, a1 = 3.00 m/s2, a2 = 9.00 m/s2.
33. A spacecraft is at elevation 125 m with velocity 9.00 m/s downward when it is decided to abort the landing and rockets fire giving it an upward acceleration of 1.50 m/s2. (a) What are the elevation and velocity of the spacecraft 10.0 s later? (b) What is the closest the spacecraft gets to the surface of the planet?
34. Most of the molecules and and atoms of the Earth's atmosphere are within 100 km of its surface. Use g = 9.65 m/s2, the mean value in this region. Ignore air resistance. (a) Find the speed of an object that falls this distance starting from rest. (b) Suppose an asteroid from space is thought to have hit the surface at 12800 m/s - determine its initial speed "hitting" Earth's atmosphere. (c) Why the difference in the "delta-v" for these two scenarios? Is disregarding air resistance justifiable in either case? Explain.
35. A physics student determines the height of a tree by timing a rock that is thrown just hard enough that it reaches as high as the tree before falling. The rock is airborne for 2.5 s. (a) How high did the rock go? (b) With what velocity was it thrown?
36. A structural engineer in an old building observes a brick fall past a window. The brick takes 0.18 seconds to pass by the 2.0 m height of the window. (a) Determine the speed of the brick at the instant it passes the bottom edge of the window. (b) The brick fell from a point how far above the top edge of the window, assuming it wasn’t thrown?
37. A great basketball player may have a vertical leap of 48 inches. (a) What “liftoff” speed, in mph, is required to leap to that height? (b) How many seconds would the player “float” in the air when jumping that high? Use g = 32.2 ft/s2
38. Determine the vertical leap of a great basketball player on the moon assuming that the initial speed is the same as that from the previous problem. On the moon g = 5.31 ft/s2.
39. An obnoxious kid in a classroom throws a pencil at the ceiling hoping it will stick like a dart. The pencil has initial speed 7.0 m/s and moves upward 2.0 m before hitting. (a) What is the impact speed of the pencil hitting the ceiling? (b) How much time is it in the air?
40. A bouncy ball is dropped from a height, h, and rebounds off the floor, returning to essentially the same height. During the actual bounce (while it is in contact) the ball is flattened and deformed by amount y before returning to its original shape. (a) Derive an expression for the acceleration, a, while the ball is in contact with the floor. (b) Use the result to calculate the acceleration if h = 1.50 m and y = 0.50 cm.
41. An object is launched straight up with a particular speed v. After an interval of time t elapses (and while the first object is still in the air), another object is launched from the same location with the same initial velocity as before. In terms of v, t, and any appropriate constants, derive and simplify expressions for: (a) the speed of each object at the instant of collision, and (b) the height at which the two objects collide.
Selected Answers
1.
a. 775 mi,
N of K-town
b. 655 mi, S
c. 105 mi, S of K-town
d. 1105 mi
2.
3.
a. 60
km, W
b. 70 km
c. 120 km/h, W
d. 140 km/h
e.
4.
a. 46 m,
439 m,
3.84 × 108 m
b. 69 yrs, 7.24 yrs,
4.36 min
5.
a. Biker A
by 19 s
b. 11 m/s
c. 0 m/s
6.
a.
b.
7. 4.68 s
8.
a.
b.
c.
9.
a. ![]()
b. 1.7 km (about 1 mi)
c. 40 km (about 25 mi)
10. a. 8.6 m/s2
b. 3.5 m/s2
c. 1.7 m/s2
d.
11. 3.8 s
12. a. 22 m/s, down
b. 20 m/s
c. 49 m/s
d.
e. t = 18.5 s,
v = 5.0 m/s
13. graph sketch
14. graph sketch
15. a. 4.1 m/s2,
S
b. 3.1 m/s2, S
c. 0.85 m/s2, S
d. 0
e. 9.6 m/s2, N
f. 5.4 m/s2
16. graph sketch
17. a. 1160 m
b. 1100 m
c. 157 m
18. graph sketch
19. a. graph
b. 1.25 m
c. 0.38 m
d.
20. a. 25 m
b. 75 m
21. a. 179.7 m/s
b. 40.14 m/s2
22. a.
b. graph sketch
c. graph sketch
c. graph sketch
23. a. 760 km/s2
(80,000 g’s!)
b. 0.54 ms
24. a. 50.0 m
b. 42.4 m/s
25. a. 9.00 m/s2,
8.91 m/s2
b. 112 m
26. 11.6 m/s
27. 25 m/s
28. a. in each case: ![]()
b. 2 graph sketches
29. a. 0.15 m
b. 0.23 m uphill of starting point
30. a. 5.45 m/s2
b. 12.1 %
c. 21.3 %
31. a. 7.6 m/s
b. 95 m/s2
c.
32. a. 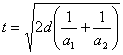
b. t = 9.43 s
33. a. 110 m, 6.00
m/s upward
b. 98 m
34. a. 1400 m/s
b. 12700 m/s
c.
35. a. 7.7 m
b. 12 m/s, up
36. a. 12.0 m/s
b. 5.3 m
37. a. 11 mph
b. 1.0 s
38. 24 ft
39. a. 3.1 m/s
b. 0.39 s
40. a.
b. 2900 m/s2
41. a. ![]()
b. ![]()